Introduction to Geographic Coordinate Systems
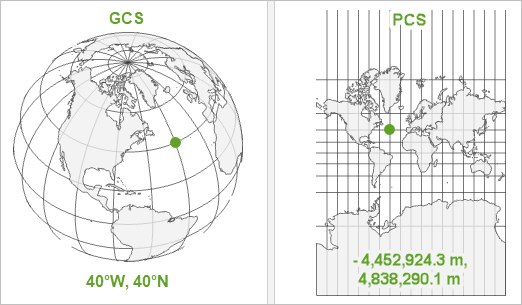
A Geographic Coordinate System (GCS) is a system that enables every location on Earth to be specified by a set of numbers or coordinates. These coordinates are usually expressed as latitude and longitude, which are angular measurements that describe a point's position on the Earth's surface. Latitude measures how far north or south a point is from the equator, while longitude measures how far east or west it is from the Prime Meridian. Together, these two coordinates allow for the precise location of any place on Earth.
The importance of the GCS cannot be overstated. It is the foundational system used in cartography, navigation, and many forms of spatial data analysis. For instance, modern GPS systems rely on the GCS to provide accurate positioning, enabling everything from car navigation systems to the tracking of wildlife via satellite. The system’s universality means that it can be used globally, making it essential for international travel, shipping, and even global climate monitoring.
Historically, the concept of a geographic coordinate system dates back to ancient Greece, with the philosopher and geographer Eratosthenes being among the first to suggest a spherical Earth and estimate its circumference. Over centuries, the system has been refined to increase accuracy, especially with the advent of modern geodesy and satellite technology. Today, the GCS is indispensable in almost every field that requires spatial understanding, from geology to urban planning.
Fundamental Concepts
The Geographic Coordinate System is grounded in a few fundamental concepts, most notably the shape of the Earth and the methods used to measure locations on its surface.
Earth as a Sphere vs. Ellipsoid: The Earth is often approximated as a sphere for simplicity in calculations. However, it is more accurately described as an ellipsoid, a slightly flattened sphere due to the planet's rotation. The flattening means that the Earth's radius is not uniform; it's larger at the equator and smaller at the poles. This ellipsoidal shape is critical for precise location measurements.
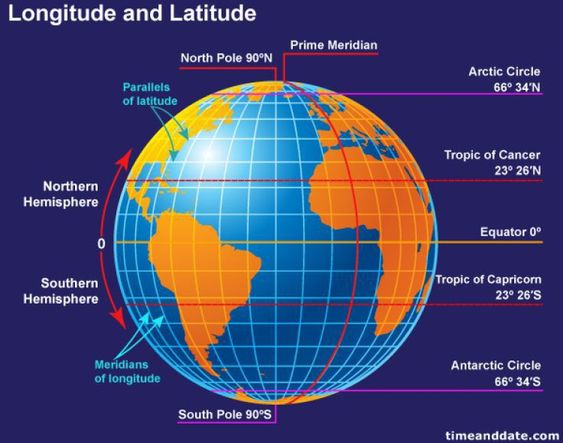
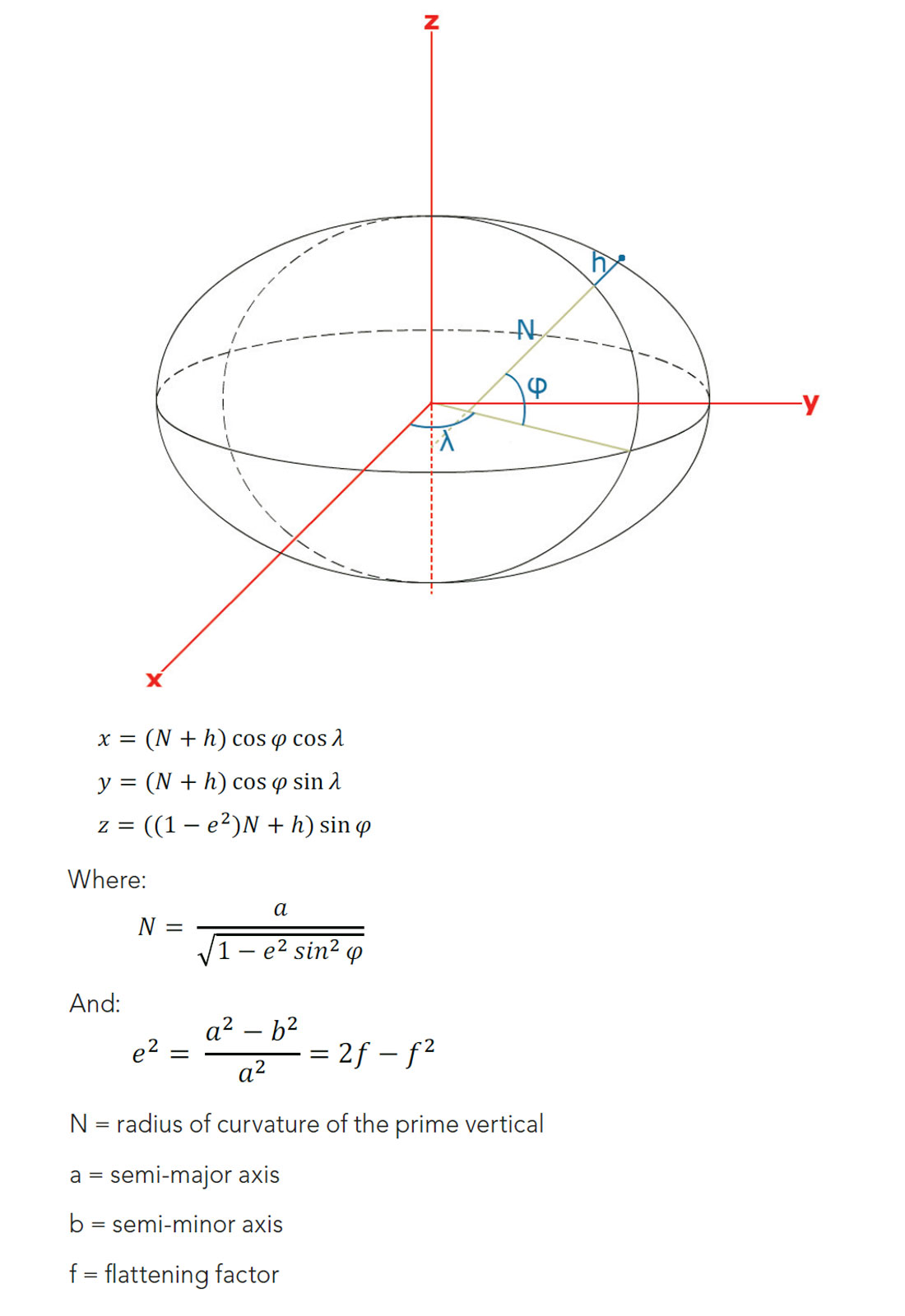
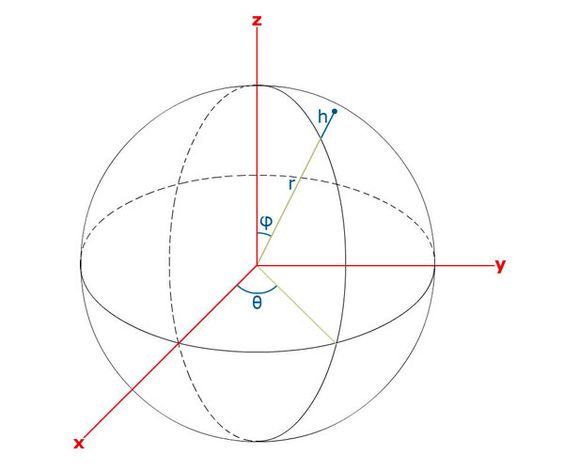
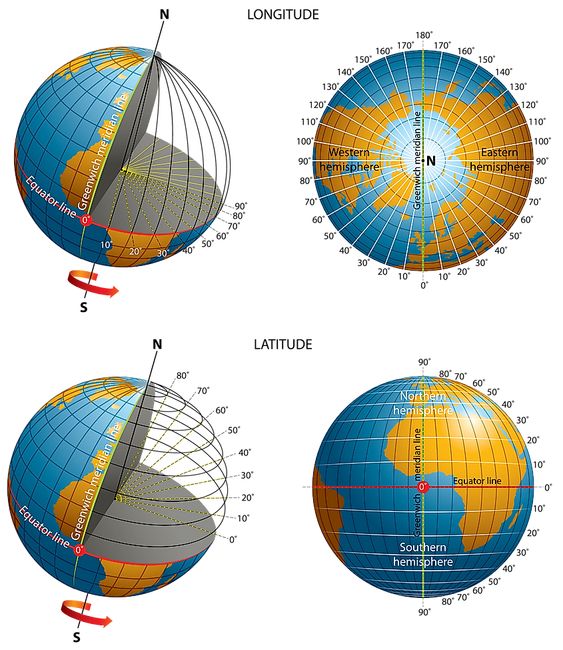
Latitude and Longitude: Latitude and longitude are the primary coordinates used in GCS. Latitude measures the distance north or south of the equator, ranging from 0° at the equator to 90° at the poles. Longitude measures the distance east or west of the Prime Meridian, ranging from 0° at the Prime Meridian to 180° at the International Date Line. These angular measurements are based on the Earth's center, making them consistent and reliable.
Prime Meridian and Equator: The Equator is the imaginary line that divides the Earth into the Northern and Southern Hemispheres. It is the reference point for latitude. The Prime Meridian, which runs through Greenwich, England, is the reference point for longitude. Together, these lines form the framework of the GCS, allowing for the establishment of a global grid system.
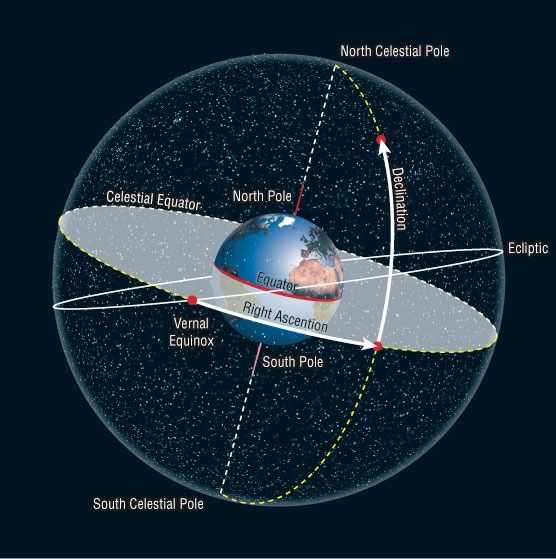
Geodetic vs. Geocentric Latitude: While latitude is usually measured relative to the equator (geodetic latitude), another type of latitude called geocentric latitude is measured relative to the Earth's center. This difference becomes significant in high-precision calculations, particularly in fields like geodesy.
Understanding these concepts is essential for anyone working with geographic data, as they form the basis of how we represent and analyze the Earth’s surface.
The Mathematical Foundations of GCS
The Geographic Coordinate System is deeply rooted in mathematical principles, particularly those related to geometry and trigonometry. This mathematical foundation ensures that the system is both accurate and consistent when applied to real-world scenarios.
Great Circles and Small Circles: A great circle is any circle that divides the Earth into two equal halves, such as the Equator or the meridians of longitude. The shortest distance between two points on the surface of a sphere lies along the arc of a great circle, making these circles fundamental in navigation and route planning. In contrast, small circles are circles on the Earth's surface that do not divide the planet into equal halves, such as the lines of latitude other than the Equator.
Degrees, Minutes, and Seconds: Latitude and longitude are usually expressed in degrees, minutes, and seconds (DMS). A degree is divided into 60 minutes, and a minute is further divided into 60 seconds. This system allows for a high degree of precision in specifying locations. For instance, the coordinates 40° 26′ 46″ N, 79° 58′ 56″ W pinpoint a very specific location, accurate to about a meter.
Conversion Between Different Units: Coordinates can also be expressed in decimal degrees, which are often used in digital systems. Conversion between DMS and decimal degrees is straightforward but essential for interoperability between different systems. For example, 40° 26′ 46″ N can be converted to 40.4461° in decimal form.
Spherical Trigonometry: Since the Earth is nearly spherical, spherical trigonometry is used to solve problems involving angles and distances on the Earth’s surface. This branch of mathematics deals with the relationships between the angles and sides of spherical triangles, which are formed by three intersecting great circles. These calculations are crucial in navigation, astronomy, and many geodetic applications.
Geodesy: Geodesy is the science of measuring and understanding the Earth's geometric shape, orientation in space, and gravity field. It provides the mathematical framework for GCS, ensuring that coordinates are accurate across the globe. Geodesy involves complex calculations to account for the Earth's irregular shape, gravitational anomalies, and tectonic movements, all of which can affect the accuracy of geographic coordinates.
Together, these mathematical foundations allow the GCS to function with the precision required for modern applications, from navigation to remote sensing.
Datums and Reference Systems
A datum is a reference point or surface against which measurements are made. In the context of Geographic Coordinate Systems, datums are crucial because they define the size and shape of the Earth and establish a reference for the position of geographic coordinates.
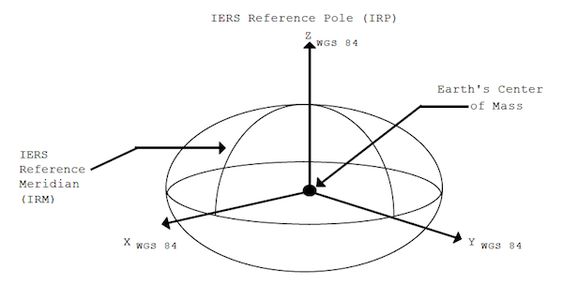
Definition and Importance of a Datum: A geodetic datum is a mathematical model of the Earth that defines the coordinate system used for geospatial measurements. It specifies the origin, orientation, and scale of the coordinate system and is the basis for calculating latitude, longitude, and elevation. The choice of datum affects the accuracy and consistency of spatial data. For example, the WGS 84 datum, used by the Global Positioning System (GPS), is a widely used global datum, providing a standard reference for geographic data worldwide.
Different Types of Datums:
- Horizontal Datums: These define positions in the horizontal plane and are used for specifying latitude and longitude. The WGS 84 (World Geodetic System 1984) is a global datum, while NAD83 (North American Datum 1983) is used primarily in North America. The differences between these datums can lead to discrepancies in location data, especially over large distances.
- Vertical Datums: These reference heights or elevations, defining how elevation is measured. Mean Sea Level (MSL) is a common vertical datum, but others exist that are tailored to specific regions or purposes.
Datum Transformations: Converting coordinates from one datum to another is often necessary when integrating data from different sources. This process, known as a datum transformation, involves mathematical equations that account for the differences between the datums. These transformations can be complex, especially when dealing with regional datums that are optimized for specific areas but may not align perfectly with global datums.
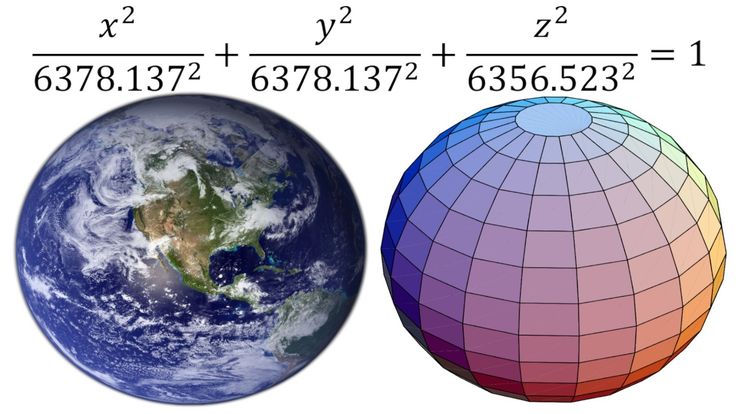
Ellipsoid Models: The Earth is modeled as an ellipsoid in most datums, which approximates its shape more accurately than a simple sphere. Different datums use different ellipsoid models, such as the WGS 84 or the GRS80 (Geodetic Reference System 1980). The choice of ellipsoid affects the accuracy of the coordinates, particularly in terms of latitude and longitude.
Datums and reference systems form the backbone of the Geographic Coordinate System. Without them, it would be impossible to consistently measure and compare geographic data across different regions and over time.
Projections and Coordinate Systems
A map projection is a systematic representation of the Earth's surface onto a flat plane, which is essential for creating maps. However, because the Earth is a three-dimensional object, projecting it onto a two-dimensional surface inevitably introduces distortions. Understanding these distortions and the different types of projections is key to effective map-making and spatial analysis.
Definition and Purpose of Projections: A map projection translates the curved surface of the Earth into a flat representation, allowing for the creation of maps. This process involves mathematical formulas that transform geographic coordinates (latitude and longitude) into planar coordinates (X, Y). The purpose of a projection is to create a usable map that accurately represents some aspect of the Earth’s surface, whether it be shape, area, distance, or direction.
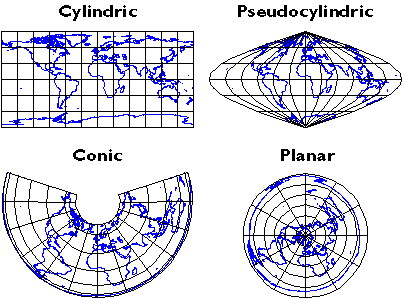
Types of Map Projections:
- Cylindrical Projections: These are created by wrapping a cylinder around the Earth and projecting its surface onto the cylinder. The most famous example is the Mercator projection, which preserves angles and shapes but distorts areas, particularly near the poles. Another example is the Transverse Mercator projection, which is used in many large-scale maps.
- Conic Projections: These are created by placing a cone over the Earth and projecting its surface onto the cone. The Albers Conic projection, for example, is used for maps of mid-latitude regions because it accurately represents area and shape over large east-west extents. The Lambert Conformal Conic projection is also popular for its ability to maintain shape and angles.
- Azimuthal Projections: These are created by projecting the Earth onto a plane, usually centered on a specific point. The Stereographic projection, which preserves angles, is often used in aviation for mapping polar regions. The Orthographic projection, which shows the Earth as it appears from space, is used for creating globes.
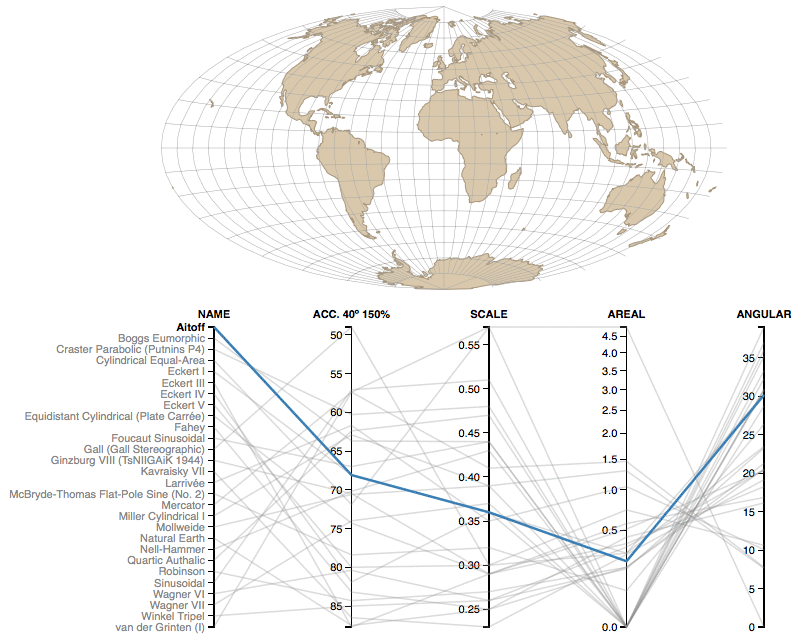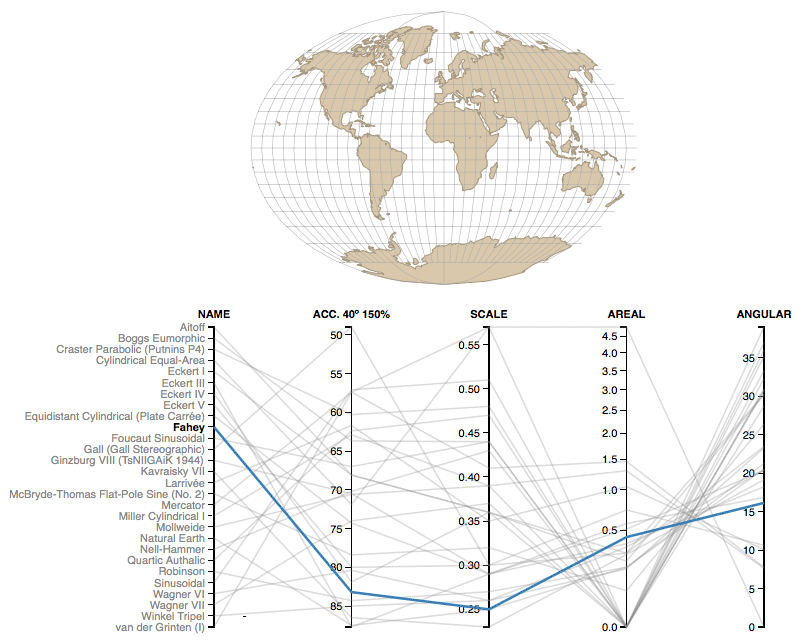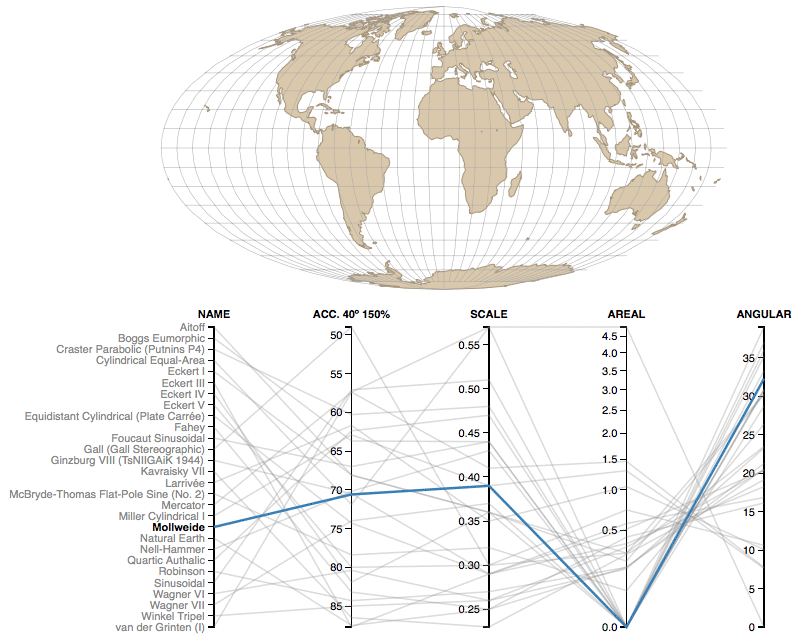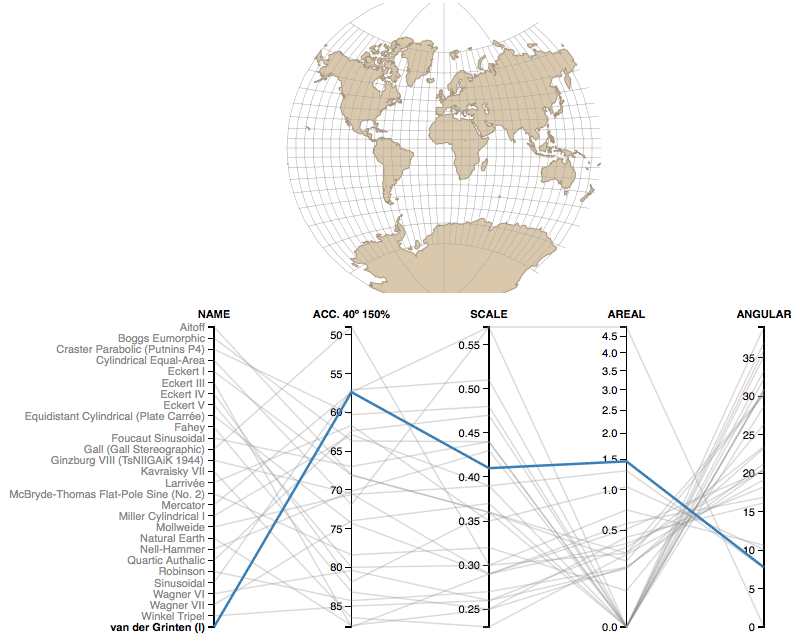
Distortion in Projections: No map projection can perfectly preserve all properties of the Earth's surface. Common types of distortion include:
- Shape: Conformal projections preserve shape but may distort size.
- Area: Equal-area projections preserve area but may distort shape.
- Distance: Equidistant projections preserve distances from a central point but may distort other properties.
- Direction: Some projections preserve direction but distort other properties, such as area or shape.
Choosing the Right Projection: The choice of projection depends on the map’s purpose. For example, if a map needs to show accurate shapes for navigation, a conformal projection like Mercator would be appropriate. For thematic maps where area comparison is important, an equal-area projection like Albers Conic might be chosen.
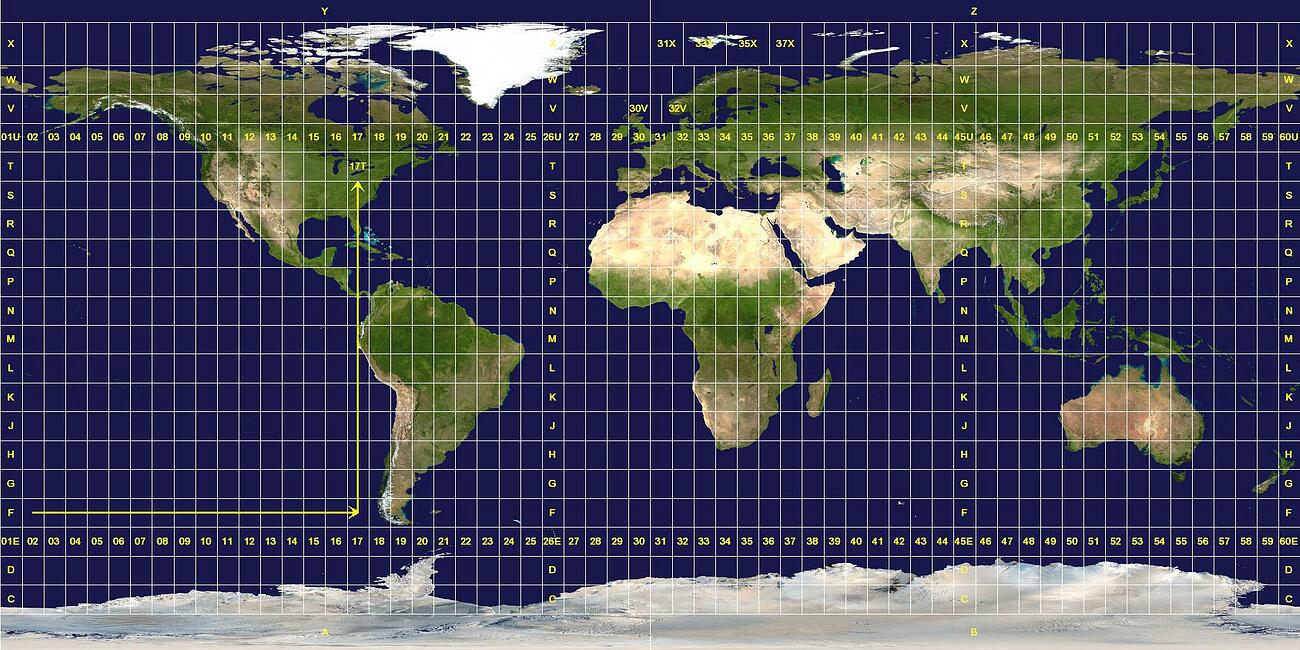
Projected Coordinate Systems: When a GCS is projected, it transforms into a projected coordinate system (PCS), which uses linear units (e.g., meters, feet) instead of angular units. The Universal Transverse Mercator (UTM) system is a widely used PCS that divides the world into a series of zones, each with its own map projection. This system is highly accurate for local and regional mapping.
Understanding projections and their associated distortions is essential for creating accurate and meaningful maps, ensuring that the chosen projection aligns with the map’s intended use.

Applications of Geographic Coordinate Systems
The Geographic Coordinate System has a wide range of applications across different fields, all of which rely on the ability to pinpoint and analyze locations on Earth’s surface with precision. Below are some of the most common and critical applications of GCS.
Navigation: One of the most direct applications of GCS is in navigation. Whether by land, sea, or air, navigating from one location to another requires accurate geographic coordinates. Modern navigation systems, such as GPS, rely heavily on GCS to provide real-time positioning data, enabling vehicles, ships, and aircraft to determine their exact location and travel along the most efficient routes. For centuries, sailors have used latitude and longitude to chart courses across the oceans, and today, pilots use similar data to guide airplanes across continents.
Geographic Information Systems (GIS): GIS is a powerful tool for managing, analyzing, and visualizing geographic data. At the heart of every GIS is a geographic coordinate system that allows for the integration of various data layers—such as terrain, population, land use, and infrastructure—into a single spatial framework. GIS applications range from urban planning and environmental management to disaster response and resource allocation. For instance, city planners use GIS to analyze traffic patterns, while environmental scientists use it to study changes in land cover over time.
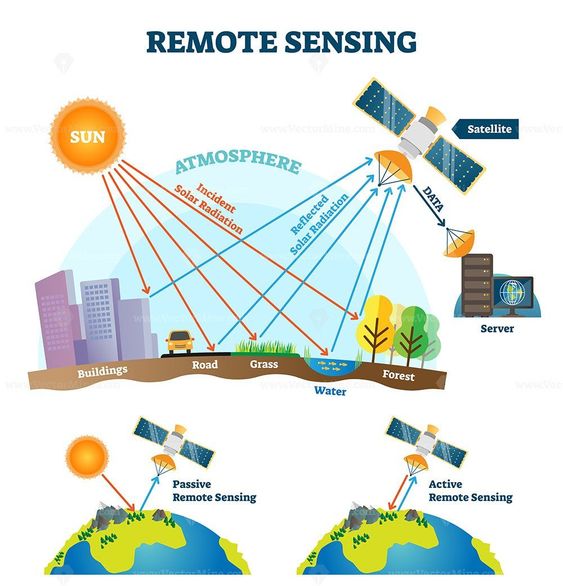
Remote Sensing and Satellite Imagery: Remote sensing involves collecting data about the Earth’s surface from a distance, typically using satellites. The data collected by these satellites is tied to specific geographic coordinates, allowing for detailed analysis of land use, vegetation cover, and other environmental factors. For example, agricultural scientists use remote sensing data to monitor crop health and predict yields, while meteorologists use it to track weather patterns and forecast storms.
Global Positioning System (GPS): GPS is a satellite-based navigation system that provides precise location and time information anywhere on Earth. GPS receivers use signals from multiple satellites to calculate their exact location in terms of latitude, longitude, and altitude. This technology has revolutionized navigation, making it possible to pinpoint locations with an accuracy of just a few meters. GPS is used in everything from everyday applications like driving directions and fitness tracking to specialized uses in surveying, aviation, and military operations.
Cartography: The art and science of map-making, known as cartography, is another field that relies heavily on GCS. Cartographers use geographic coordinates to create maps that represent the Earth’s surface accurately. Whether it’s a political map showing country borders or a topographic map depicting elevation changes, GCS ensures that the features on the map correspond to their real-world locations. Modern cartography also involves the use of digital tools, which allow for the creation of interactive and highly detailed maps.
Surveying: Surveyors use GCS to determine the exact location of points on the Earth’s surface, which is crucial for land ownership, construction projects, and infrastructure development. Surveying involves measuring distances, angles, and elevations, all of which are tied back to a geographic coordinate system. For example, before a new building is constructed, surveyors must establish the precise boundaries of the property and the location of key features such as roads and utilities.
In all these applications, the Geographic Coordinate System provides a common framework that allows for the consistent and accurate representation of location data, making it an indispensable tool in modern science, technology, and daily life.
Challenges and Advanced Concepts
While the Geographic Coordinate System is robust and widely used, it also faces several challenges and involves advanced concepts that must be understood for accurate application, especially in high-precision contexts.
Coordinate System Accuracy: The accuracy of geographic coordinates depends on several factors, including the precision of the measuring instruments, the quality of the reference datum, and the method of measurement. In many applications, even small inaccuracies can have significant consequences. For example, in land surveying, an error of just a few centimeters can lead to legal disputes over property boundaries. GPS technology has significantly improved coordinate accuracy, but factors like satellite geometry, atmospheric conditions, and multipath effects (where signals reflect off surfaces) can still introduce errors.
Datum Shifts and Plate Tectonics: The Earth’s surface is dynamic, with tectonic plates constantly moving. This movement can cause shifts in the positions of geographic coordinates over time. For example, the Pacific Plate is moving northwest at a rate of several centimeters per year, which means that locations on this plate are gradually shifting relative to the fixed coordinates of a global datum like WGS 84. To account for this, some regions use dynamic datums that are updated periodically to reflect these movements. In addition, when switching between different datums, coordinates may need to be transformed to maintain accuracy, which can be a complex process.
Time-Dependent Coordinates: In fields like geodesy and GPS, it is sometimes necessary to consider the time-dependent nature of coordinates. As the Earth’s crust moves, the positions of fixed points (such as survey markers) change over time. This means that a location’s coordinates can be different depending on the time they were measured. Time-dependent coordinate systems account for these changes by incorporating a temporal component into the coordinates, allowing for more accurate tracking of positions over time.
Global vs. Local Systems: Global coordinate systems, like WGS 84, are designed to be used anywhere on Earth, providing a consistent framework for global applications like satellite navigation. However, in some cases, local coordinate systems are more appropriate. These systems are optimized for specific regions and can provide higher accuracy than global systems by taking into account local variations in the Earth’s shape and gravity field. The challenge lies in integrating data from different systems, which often requires complex transformations to ensure consistency.
Three-Dimensional Coordinate Systems: While traditional GCS deals with latitude and longitude (2D coordinates), many applications require the incorporation of elevation to create a three-dimensional coordinate system. This is particularly important in fields like aviation, where altitude is a critical factor, or in 3D mapping and modeling, where terrain and built structures need to be represented accurately. 3D coordinates are usually expressed as (latitude, longitude, elevation), but integrating this third dimension introduces additional complexities, such as the need for accurate vertical datums and consideration of the Earth’s curvature.
These challenges and advanced concepts illustrate that while the Geographic Coordinate System is a powerful tool, its application requires careful consideration of various factors to ensure accuracy and reliability, especially in high-precision or dynamic environments.
Practical Implementation and Case Studies
To fully understand the Geographic Coordinate System's impact, it’s useful to explore practical implementations and real-world case studies that demonstrate its versatility and importance across different domains.
Case Studies in GCS Applications:
- Disaster Management: Geographic coordinates are crucial in disaster management, where they help responders pinpoint affected areas, assess damage, and coordinate rescue operations. For example, during the 2010 Haiti earthquake, GPS technology was used extensively to map the disaster zone, guide relief efforts, and plan the reconstruction. Accurate geographic data allowed responders to quickly identify the most affected areas and deploy resources efficiently.
- Climate Science: GCS plays a significant role in climate science, where it is used to track changes in environmental conditions over time. Satellite data, tied to specific geographic coordinates, enables scientists to monitor phenomena like deforestation, glacier retreat, and sea-level rise. For instance, the Landsat program, which has been collecting satellite imagery since the 1970s, provides a long-term record of Earth’s surface, helping scientists understand the impacts of climate change.
- Urban Planning: In urban planning, GCS is used to analyze spatial data related to land use, infrastructure, and population distribution. Planners use this information to make decisions about where to build new roads, schools, and housing developments. A case study from New York City illustrates how GCS-based GIS was used to identify areas with poor access to public transportation, leading to targeted improvements in bus routes and subway stations.
GCS in Disaster Management: In disaster management, GCS enables the precise location of hazards, vulnerable populations, and critical infrastructure. This precision is essential for developing effective emergency response plans. For example, during hurricanes, the National Hurricane Center uses geographic coordinates to track the storm’s path and issue warnings to affected areas. Similarly, during wildfires, firefighters use GPS coordinates to map the fire’s perimeter and strategize containment efforts.
GCS in Climate Science: Climate scientists rely on geographic coordinates to monitor and model environmental changes. By analyzing satellite data with precise geographic coordinates, they can detect trends such as shifts in vegetation patterns, the spread of deserts, and changes in polar ice coverage. These observations are critical for understanding the long-term impacts of climate change and informing policy decisions aimed at mitigating its effects.
GCS in Urban Planning: Urban planners use GCS to analyze the spatial distribution of populations, infrastructure, and services. This information helps them design cities that are more efficient, sustainable, and livable. For example, by mapping the locations of schools, hospitals, and parks, planners can identify underserved areas and prioritize them for development. GCS also aids in traffic management by allowing planners to analyze patterns of movement and optimize road networks to reduce congestion.
Through these examples, it's clear that the Geographic Coordinate System is more than just a theoretical concept; it is a practical tool with wide-ranging applications that have a profound impact on society.
The Geographic Coordinate System is a cornerstone of modern geospatial science, enabling us to describe, analyze, and interact with the Earth’s surface in a precise and standardized manner. From its mathematical foundations to its practical applications, GCS is essential for a wide range of activities, from navigating our world to understanding complex environmental changes. As technology advances and our need for accurate spatial information grows, the importance of GCS will only increase, underscoring its role as an indispensable tool in the geospatial sciences.
In conclusion, the Geographic Coordinate System is not just a tool for cartographers and surveyors; it is a vital component of our daily lives, embedded in the technologies we rely on, the decisions we make, and the ways we interact with the world around us. Whether we are navigating through unfamiliar terrain, managing natural disasters, or planning the cities of the future, the Geographic Coordinate System provides the framework that makes it all possible.